Introduction

This curriculum unit is on symmetry and fractals with an emphasis on fractal structures in the lung. I urge that there is a way to ensure that geometry concepts diffuse the mathematics environment while still engaging to students. I have tried to come up with lessons that will help teachers realize strategies to teach geometry to their students. It is my hope that completing this curriculum unit will aid teachers to teach fractals in a way that will motivate students, assist their connection and approach of the "real world" situations to the concepts, aid their use of different strategies, and extend students' skills to solve math problems in other situations.

I teach in a school district with approximately 24,000 students. They are 88% African American, and 80% are eligible for free or reduced lunch. I teach mathematics to 7 ^th and Pre-Algebra students. The unit can be used, with some modifications to the activities, by higher level grades as well. In my situation, the unit will be taught over approximately 2 weeks for 90 minutes each day. I teach in under-resourced, urban areas and my students come with various educational needs. Some of these needs can be easily changed in the classroom, some cannot. Since NCLB there is not much that matters except for their standardized test scores at the end of each school year. If students meet standards according to state test, schools are viewed as successful and the stress in "passing the test" is put off for another year.

The students I receive each year must have some prerequisite skills in describing and implementing symmetry. Usually, they don't. Most of the time, there is a great difference between what the students need to know to "get started" and what they actually know. Of course, I have to begin my instruction "where they are." This means that I do not have the advantage of merely working on the concepts and strategies. I have to start my students off with the elemental parts of geometry, nature and shapes. If they master these skills, I need to teach them how to approach geometry in an investigative manner using such techniques as collaborative learning; analysis and problem solving to express, test, and locally confirm or disconfirm conclusions; written and oral assignments to establish useful communication skills; and tools such as representations, and application software.

The unit will scope concepts of geometry starting with types of symmetry. That will give a starting point for mathematical relevance in the real world. In our case, we will consider the lungs. We will then examine fractals and, in particular, the fractals that exist in the lungs. If time permits, we will consider a final project. With this project, the students will design and build a prototype of an artificial lung. The reason for this project is for them to apply the knowledge they already know in ratio and proportion, symmetry, fractals, scale values and area.

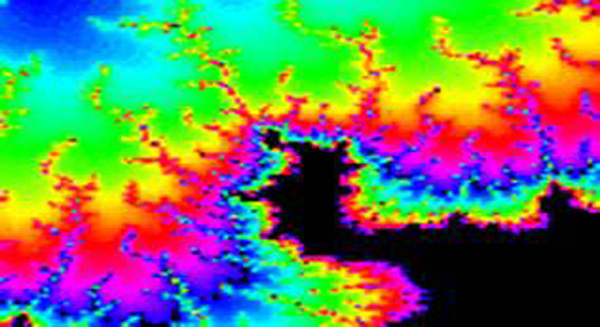
Figure 1 Coastline of Europe

Fractals have really interesting ideas connected to them. Imagine that the image in Figure 1 is a representation of the coastline of Europe. Should you trail it with a mile-long ruler you will get a satisfied measurement. Now, let's say you come back the next day to measure it with a meter-long ruler, should you get another satisfied measurement? Which of the two measurements would give you a larger measurement? Since the coastline is jagged, you could get into corners and crevices better with the meter-long ruler, so it would yield a much satisfied measurement. What if someone decides to use an inch-long ruler to measure it? They could really get into the smallest and insignificant of fissures there. The analysis will get bulky since we know that the coastline is broken at a scale smaller than an inch. What if it were broken at every point on the coastline? You could analyze it with smaller and smaller tools, and the analysis would get longer and longer. That's an example of a fractal design. Therefore, the length trait of a fractal design depends on the scale at which you analyze it.

Most mathematics we teach in school today is old knowledge. For example, the study of the geometric shapes was established around 300 B.C. by Euclid. On the other hand, fractal geometry is newer and that research is still underway to prove some aspect of it. In the meantime, there is a lot we can learn and understand about fractals. A lot of objects around us are not made with your basic geometric shapes like squares or triangle, but are made with much more complex ones. For example things like ferns and snowflakes have fractal attributes that people use to solve real- world problems. Engineers are using the knowledge in developing and building fractals to solve engineering problems.

One of the main concepts of geometry, especially advanced geometry, is the notion of sound logic and proof. In an attempt to show students how to relate what we learn in the classroom to our everyday lives and environments, we will look at a very simple everyday item like the human lung. We will look at the development of the lungs, paying particular attention to the fractals that exists within it. We will also be looking at the lungs because it is the one thing that the students do study in their science classes. Studying them in a math class will strengthen their understanding, and will help them realize that the two subjects, math and science, truly do move hand in hand. We will look at the mathematics in the branching of the lungs, the type of branching in them, and calculate their fractal dimensions. We will accomplish this in two approaches. One, by manually calculating the dimensions and two, by using computer generated software. This will enable the students to see that mathematics does not always need to be done with paper and pencil.

Computers have become a great resource in our educational system these days. Now, most of the state standardized tests are taken online. It is crucial that we make strides in preparing our students for the hurdles introduced by new technology. I am a crusader in teaching students with technology. Research shows K to 12 students spend about 75% of their time on some type of computer-aided program: playing computer games, video chatting on the computer, downloading and uploading podcasts, etc. So it is imperative that, as educators, we use these avenues to our advantage as well. Also, most of my students become excited and focused anytime an activity or lesson is being done using computer aided software. In this regard, as I teach this unit, I intend to show them how to use computer programs to achieve the same results they achieved manually or with the aid of a calculator. They will be shown how to calculate fractal dimension using a computer-aided program like Winfeed.

Rationale

A lot of teachers, including myself, do not take class time to teach students about fractals. More often than not, I will just mention it and give a few examples of it. Now, when you look around us and also take a look at the visual arts and sciences, you will realize that fractals are present. Also, as recommended by NCTM connections and standards, mathematics teachers should connect the math to their students' environment. So another way that I will accomplish this is to teach fractals in relation to the human organ. Fractal geometry is a really "cool" concept to teach. They change the way test, classify define, and evaluate the world around us, and opens new doors in the world of mathematics.Fractal images are very mysterious and students tend to get very inquisitive and excited to try to find out how these images come about. Another exciting fact about fractals is, you do not have to be a genius to take them on.

Connecting mathematics and the sciences has been one of the main objectives of the country's educational system. This unit will be used to bridge further the gap between mathematics and science by looking at symmetry, fractals and the lung. Someone will say "the lungs…what has that got to do with mathematics?" Geometry is an important aspect of many of the standardized tests. On standardized tests, the concepts are often not so straightforward, but are hidden within other concepts. Some students will automatically give up when they see such questions. Others that know the concept have a difficult time trying to relate it to other concepts and hence are not able to answer the questions. By developing the confidence and the skills to tackle fractals, students will not only improve their math skills with the four basic operations, but will also hopefully transfer their knowledge to other mathematical areas.

Fractals can be seen all around us, there is the need to take some time off to teach these concepts and their properties. Several articles in mathematical journals, written by professionals in the field like, Cynthia Laniu of Rice University, also express the need for teaching fractals and its properties as a part of the math curriculum. In lieu of this I will use this unit to showcase how we can achieve this.

Objectives

Prior to researching and writing this unit, I did not understand many of the concepts that are described here. Now, I understand these concepts, and I am able to explain and relate them to myself on numerous levels. This is the objective of this unit: that any teacher can take this unit, understand it, tweak it to suit the grade level he/she teaches, and impart the knowledge to their students. My other objective is to be able to use this unit to get students to understand geometry and fractals, while having fun with the concepts.

Background

What is a Fractal?

Fractals are shapes that can be broken down into smaller shapes, where each small shape resembles the original shape. This property is also called self-similarity. Fractals originated in the 17 ^th century with studies by Karl Weierstrass, Georg Cantor, and Felix Hausdroff. It is said that Benoit Mandelbrot came up with the term fractal in 1975. It came from the latin word fractus meaning "uneven." Fractals as defined by Webster's Dictionary is "irregular shapes for which any suitably chosen part is similar in shape to a given larger or smaller part when magnified or reduced to the same size". Fractals are useful in a lot of areas. These include, but not limited to, medicine, soil mechanics, and technical analysis.

Characteristics

True fractals often have clear systems at endlessly small scales. This is a bit difficult to explain using geometric language. Basic fractals are self similar and due to this, they are said to be immeasurably complex. They also have a simple and repetitive definition.. Fractal generating software can be also be used to generate fractals. These types of computer generated fractals are not considered as real fractals because they do not always appear to have the same attributes as real fractals. Examples of some real fractals are coastlines, snowflakes, and patterns of some flowers, clouds, mountains and fabric.

Generating Fractals

In Math, fractals can be separated according to the way they are generated. There are four ways of generating fractals. Iterated Function Systems, IFS, is the most basic one. IFS structures show self similarity, which means that their attributes at any magnification are the same as the whole. Then there are Escape-Time Fractals. These come about by a formula relation at every point in its space. An example of this type of fractal is the Mandelbrot set. Random Fractals and Strange Attractors are the remaining types of fractals. These fractals are accomplished from complex systems. They cannot be explained mathematically and are out of the scope of this curriculum unit.

Iterated Function Systems

Iterated Function Systems is a form of generating fractals that always ends up with a self similar fractal. This type of fractal was studied extensively by John E. Hutchinson in the eighties. These fractals appear in a number of dimensions but are normally initiated and etched in two dimensions. One obvious attribute of an IFS fractal is that they are normally made up of copies of themselves whereby each copy is transformed by a function system. The work of these functions is to gather all the individual points together to make smaller shapes. That is the reason why IFS fractals always seem to consist of smaller sizes of the original shape. Examples of some IFS fractals are Sierpinski's Gasket, Cantor sets and Koch's Snowflake.. In Michael Barnsley's his book "Fractals Everywhere", he said that "IFSs provide models for certain plants leaves and ferns by virtue of self-similarity which often occurs in branching structures in nature."

Escape-Time Fractals

This type of approach in developing fractals can also be known as round fractals. They are called round fractals because these types of fractals seem to be orbiting endlessly. Typical examples are the Mandelbrot set and the Julia sets. Escape time fractals are not that difficult to generate. To generate them, an algorithm is applied to every point in a complex plane. Then a unique color is assigned to the value whether or not it approaches infinity.

Random Fractals

This form of developing fractals is fairly candid. Just as the name suggest, random fractals are developed by random processes instead of determined processes. This means that even though its starting point is known, there are many possibilities the process or path might go to. Sometimes the process or path might be predictable and sometimes not so much. A perfect example of this kind of fractal can be seen when you look at coastlines on a map or atlas.

Strange Attractors

We can never talk about strange attractors without talking a little about chaos theory. In chaos theory, changing systems, with the help of some minute initial changes, will over time, lead to large unpredictable results. If anyone has seen the movie titled 'The Butterfly Effect" with Ashton Kutcher, this same principle was used as the plot of the movie. In the movie, Ashton played a 20 year old student who realizes that he has the ability to travel through time to his former self and make some changes which eventually results in changes in his present life.

With that said, in strange attractors, a series of disorderly branching of a system, say fluid flow, results in an attractor. This attractor is known as a strange attractor because they do not have unit dimensions. The directions in some strange attractors can be characterized and some not so much. A common example is the Lorenz attractor.

Classifying Fractals

Fractals can be classified using different attributes. The most efficient way of grouping fractals will be to look at the properties of the kind of self similarity they exhibit. Self similarities exist in three different ways which are exact, quasi and statistical self similarity.

Exact self similarity fractal

Just as the name suggests, the fractals appear exactly the same. They just appear in smaller sizes than the previous shape. A typical example will be in an Iterated Function System, like the Sierpinski's Triangle, after the main triangle, the same triangle appears over and over again. It just gets smaller and smaller each time.

Quasi self similar fractal

The word quasi simply means almost. That said, these kind appears quasi but not exactly the same as the original even though they do appear in smaller sizes than the previous shapes. Example, if you zoom into a Mandelbrot set, you will see that in each resulting shape, the shape is not as oval shaped as its predecessor. This type of fractal is called the almost (quasi) self similar fractal.

Statistical self similar fractal

These fractals have values which are maintained across distinct proportions. That is why random fractals are put under this kind. Also in type, the fractals do not have an equal distortion between shapes. Instead there is a value which is maintained across the scales. A typical example is the coastline of Britain. One does not expect to see little miniature versions of it coming together to create it by observing it under a microscope.

The human lung

The lung is a crucial organ in mammals for inhaling and exhaling of oxygen and carbon dioxide respectively. It can be found on either side of the heart. The lung serves as the pathway for oxygen to move from the atmosphere into the blood and carbon dioxide to move into the atmosphere from the blood. This exchange of gases is made possible by alveoli. Before the exchange of gases happens in the alveoli, the air has to first go through the nose or mouth, then through the larynx, trachea, and a system of bronchi and bronchioles. The lungs look like a waxy structure that contains adjacent hexagonal cells filled with epithelium. The lungs in mammals might look smaller when you look at its outer surface area but in actuality, it is much larger. This is due to the fact that lungs grow as branching fractals. That is, lungs develop without covering a large surface area. Human lungs are divided into two fragments, one on each side of the heart. The right lung has 3 sections while the left has 2. These sections are further divided into subsections called lobules. We will take a closer look at the lungs in detail in the strategies.

Strategies

I will begin teaching the unit by introducing basic geometry, that is, symmetry. We will review symmetry and the different types of symmetry that exist. Then we will delve into fractals, with emphasis on the Iterated Function System (IFS) formalism for generating fractals. I will also talk about the application and examples of IFSs. In an effort to bridge the gap between mathematics and the real world, i.e. science, we will take particular look at an example of a human organ, the lung, which almost all of them are be familiar with by this time. I will also make sure that the students have studied about the lungs in their science class. This will make teaching the unit easier for me, and more satisfying for the students. First and foremost, we will talk about symmetries in nature. This will segue into our topic of fractals and the symmetries that exist in them.

There are three forms of symmetry we are familiar with. These are translational, reflectional, and rotational symmetry. Translational Symmetry is when a shape is displaced either horizontally or vertically. An example is a brick wall. Reflectional Symmetry is when a geometric shape can be split into two parts by a line, one half of the shape removed and the missing piece can be replaced by a reflection of the remaining piece. A perfect example is the human face. Rotational Symmetry is when a geometric shape is rotated about some point and its resulting image is the same as the original geometric shape. A common example is the geometric shape of a snowflake. Rotating it by 60 degrees will return the shape to its original shape.

After going over the basics of symmetry, we will take a look at the geometric attributes of simple fractals. In other words I will use the concept of self similarity to explain simple fractals.

Now that the students have reviewed symmetry and know what fractals are, they are ready to look at a mammalian organ, the lung, to see if there exist any forms of symmetry.

Symmetry in the Lungs

You can find some existence of symmetry between the right and left fragments of the lungs. Figure 2 shows how the right and the left lungs are subdivided into lobes. Each subdivision in the lobe is called segments. The right lobe has 10 segments. That is, 3 in the upper part, 2 in the middle part and 5 in the lower part of the right lobe. Just as the right lobe has segments, so does the left lobe. The left lobe has 8 segments. That is, both the upper and lower left lobe contains 4 segments. The diagram clearly labels the names of each of the segments in both the left and right lobes. The lobes are covered by the inner pleura, which is in contact with the outer pleura as it reflects from the lateral surfaces of the mediastinum. The inner pleura form introversions into both lungs, which are called fissures. These fissures are used to keep both right and left lungs apart and to do so, both lungs have 2 fissures. One of the fissures in the left lung, however, is incomplete.

Figure 2 the Human lung

The closest of the symmetries that exists in mammalian lungs is reflectional symmetry. As a quick classroom hands-on activity, we will use Geometer's sketch pad to make a sketch of the lungs. The goal here is to let the students become familiar with the organ that they are about to study. They will use the tools in Geometer's sketch pad to make copies of the lung sketches and position them in creative patterns. Now I will begin to introduce to the students fractals: both what they are and how to generate them. "I find the ideas in the fractals, both as a body of knowledge and as a metaphor, an incredibly important way of looking at the world." Vice President and Nobel Laureate Al Gore, New York Times, Wednesday, June 21, 2000, discussing some of the "big think" questions that intrigue him.

Generating Fractals with emphasis on Iterated Function System (IFS)

Fractals can be created in a variety of ways. One way is IFS, defined above, whereby the resulting fractal is self-similar.-. This unit will focus on drawing and computing fractals in 2D. Two dimensional (2D) fractals consist of copies of the original shape being altered by a function. A perfect example is the Sierpinski triangle. The functions make the shape smaller by connecting the points. Therefore the shape of an IFS fractal is made up of an assembly of smaller copies of itself, with each smaller copy also having an assembly of smaller copies of itself to infinity. This makes it a self-similar fractal.

Wac?aw Sierpi?ski's triangle is one of the simplest of geometric images. His triangle may also be constructed using a predictable algorithm. Predictable algorithm in the sense that we know what the output is supposed to resemble and hence given any size triangle, we can still produce the Sierpinski Triangle. To see this, we begin with any size triangle. Then we find the center of each side and use it as the tip of a new triangle, which we then cut out from the original triangle. What we are left with here, are three triangles each having an area which is exactly a quarter of the original and whose dimensions are one-half of the original triangle. Also, all the resulting triangles we created look exactly like the original. This process can ne continued to infinity and the same copy of the triangle will be created, it just gets smaller and smaller. That is, from each remaining triangle we remove the "middle" leaving behind three smaller triangles each of which has dimensions one-half of those of the parent triangle (and one-fourth of the original triangle). So should you just follow the process, for say 2 stages, clearly 9 triangles will remain at this stage. At the next iteration, 27 small triangles, then 81, and, at the Nth stage, 3 ^N small triangles remain. See figure 2 below.

At this point some students will ask, why do the triangles appear in powers of 3? The explanation will be because a triangle has only three sides and hence when we iterated, we got 3 smaller triangles from the original triangle. Should we have used a different shape that ended up giving us a different number of iteration other than 3 from its original, it would have been in powers of that number.

It is easy to check that the dimensions of the triangles that remain after the Nth iteration are exactly (1/3) ^N of the original dimensions (Figure 2).

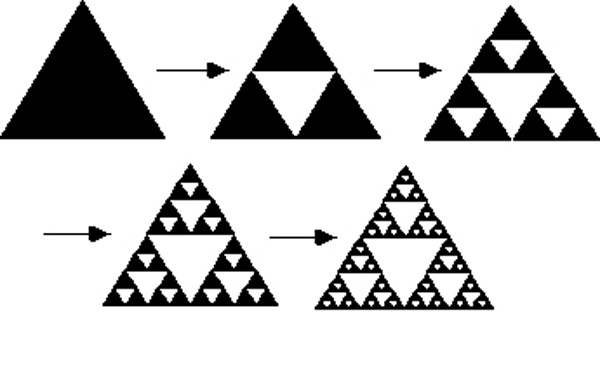
Figure 3 Deterministic construction of Sierpinski's Triangle

Now, to help train the students' eyes to find fractal decompositions of objects, I will let them try to find smaller copies of each shape within the shape. I will show pictures of a tree, a snowflake, a lace, and or a fern and ask them to practice.

The concept used in Sierpinski's triangle can be used, with some changes, to develop other fractals that exist in nature. Look at the creation of the snowflake below. It is made from drawing six lines from the middle to their borders. Then two lines are drawn, this time, from each line. Continuing this process to infinity will leave you with a pretty picture like the one in figure 4 below.

Figure 4 Creating a snowflake using fractals

Initiators and Generators

Now that the students know how to find similar shapes within a shape, they can be introduced to the right process within self-similarity. Self-similarity is an elaborate way of saying to repeat a process over and over again. One way to realize self-similarity is to build the same shape within a shape and duplicate this algorithm infinite number of times. Each time realizing how the shape and the area being covered get smaller and smaller. This phenomenon is accomplished by two means. The initial action is the initiator and the second action is the generator. The initiator is the name given to the first item or original shape and the generator is the second and subsequent items. To realize this, let us look at figure 4 above. Here we are building a flake using the idea of initiators and generators. The initiator here is the 6 lines we drew from the center to the edge. While the two lines we drew over and over again, on the lines, are our generators. Therefore the key is this, substitute an image of the initiator with a magnified image of the generator making sure to indicate the direction where possible.

Measuring Fractals

The students in higher level classes should be ready at this point to move on to measuring fractals. There are a variety of procedures out there for measuring fractals. One effective way that I will introduce to my students is called the self-similarity dimension. Self-similarity dimension can only be used to measure fractals if the fractals posses self-similarity properties.

So if a fractal possesses self-similar property, then we can represent the number of self-similar pieces by a letter, say N. Lets also say these self-similar pieces increase by a scaling factor, say l. Finally, let us represent the dimension by a letter, say D. From the explanation of self-similarity above, we all agree that the scaling factor, l, increases exponentially. Therefore, it is safe to say that N =l ^D. Taking logarithm of both sides, we get, D =(log(n)/log(t)). Teachers of higher level math will be able to continue on with more complex shapes where the knowledge of logarithms will be useful.

There is a computer program called Winfeed which is a fractal examination software. With this program, the user is able to examine functional emphasis, including Mandelbrot and Julia sets, ferns and snowflakes, web and bifurcation diagrams, and more. With this program, all one has to do is upload the picture you are trying to calculate. Then hit a button and it automatically calculates the properties.

At this point, the students are better equipped to take on the development of fractal geometry in the lung. We will talk about the lung, its anatomy and physiology. Then look at an outline of the lungs and try to identify what type of symmetry exists there. Eventually, we will evaluate and measure the fractals that exist in them.

The fractal development of the lung

We already know that fractals help lungs develop. This means that lung lobes develop by splitting into smaller lobules. So the question to the students at this point is do the lungs continue splitting? Do they continue splitting to infinity? Is there an actual point when they stop splitting? How many lobules or smaller magnifications can there be? To answer these questions, we will look at the development of the human lung.

The development of a human lung is very confusing. It is broken into phases or stages. The first phase is the embryonic phase. In this phase, bronchial tree is laid out and the lung segments are defined. The next phase is the glandular or pseudo glandular phase. In this phase, bronchioles are formed. The next phases are the canalicular phase and the saccular phase. Branching and splitting occurs mainly in the embryonic phase and hence that is where our focus will be as a class.

Figure 5 below shows the generation of the lung in the embryonic phase. It shows how branching at each generation phase. Let us give the generation phase a letter, say z. Since the branching of the bronchi and the notion of self-similarity are the same, we will not be wrong to say that the branching increases exponentially as well. If so then we can say at each generation phase, z, we get 2 ^z branches. In our lungs however, we can observe branches up to about 2 ^{2 3}

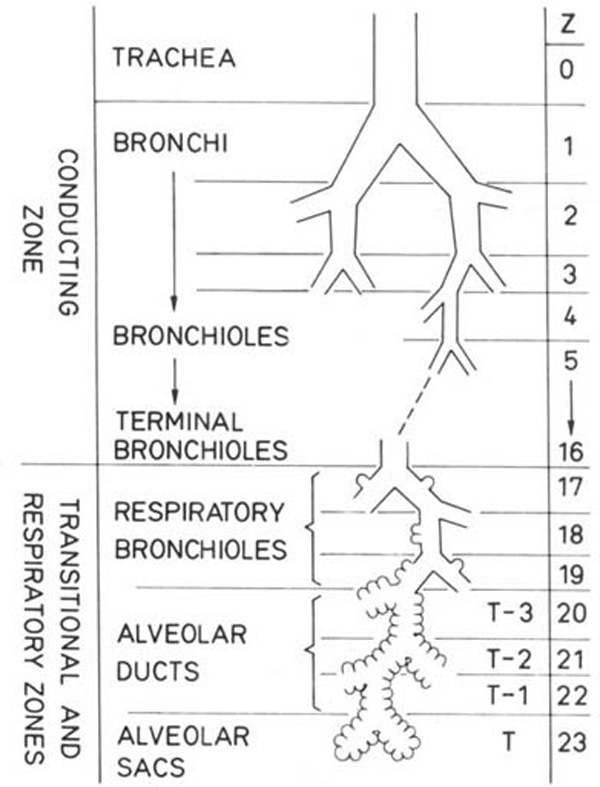
Figure 5. Fractal development in the lung

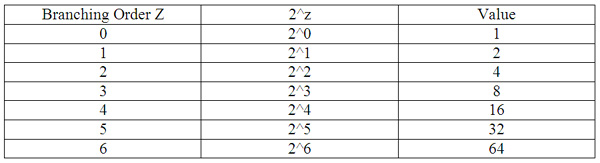
As the lung keeps branching and splitting from 0 to 1 to 2 and so on, it is doing so, in this case, in base two. Hence, 2 ⁰ = 1, 2 ¹ = 2, 2 ²= 4, and so on. This is also termed bifurcation. Bifurcation therefore is the separating of a body into two parts. In a human lung, the division continues up to a power of 23. That is 2 ^{2 3} as seen in figure above. This is where the students will use their knowledge in powers and exponents to create a table of powers, so to speak. The table will be similar to the table below. The students will compute this manually all the way up to 2 ^{2 3}.

Importance of fractals in the lungs

Just as fractals can create magnificent pictures and designs so are they important in our lives. The existence of fractals in the lungs not only compresses the size of our lungs but also protect us from physical stress while they grow. Due to the compact nature of our lungs, thanks to fractals, our lungs are more efficient. The alveoli are the little air sacs in the lungs whose main task is to circulate oxygen in the blood. The time at which the circulation happens in the alveoli is directly proportional to the area of the alveoli in the lungs. Research shows that the surface area of an adult mammalian lung is750 sq ft and still they have a small volume.

As a whole, the lungs show properties of fractal geometry. Also, in the bronchi and bronchiole tubes, the property of bifurcation can be observed.

Activity 1

In this activity you will be creating a dragon using strips of paper. You will be folding the strip of paper as many times as possible following a particular pattern. You will be filling out a chart as you go along as well and answer the questions that follow.

Materials/Resources:

Paper strips, chart, calculator

Directions/Procedure

1: Start with a strip of paper (one segment). Using a marker, place a dot in the left corner. Keep the dot facing down in left hand for each stage.

2: Fold the strip of paper in half. Unfold and form a right angle with the crease. Record how many equal segments were created and how many creases(folds) were made on chart.

3: Refold the segment so it is in ½ again. Now fold in ½ a second time. Unfold and form right angles at the creases. Record the number of segments and creases on chart.

4 and 5: Repeat the steps in 2. Record segments and creases on chart.

Fill out the chart as you create your paper dragon and then answer the questions using the data gathered.

1. Going down the column of segments, what pattern do you notice? Fill in the rest of the column.

2. Going down the column of creases, what pattern do you notice? Fill in the rest of the column.

Challenge Questions:

1. What equation can be used to find the number of segments for 25 folds? How many segments will there be?

2. What equation can be used to find the number of creases for 15 folds? How many creases will there be?

Activity 2

In this activity you will be using a computer program called Winfeed to find fractal dimensions of lungs.

Materials/Resources:

Computer, Winfeed software, Scanner, pictures of lungs

Directions:

1: Take your students to the science lab in your school or take a field trip to the nearest science lab and take pictures of the lungs of different dissected animals.

2: Help students scan and save the pictures onto a computer.

3: Make sure you have downloaded and installed the Winfeed Software on all computers. The software can be found online for free and it takes just minutes to install.

4: Open Winfeed and click window in the top menu. Click dimension in the drop down menu. A window named noname1 opens at this point. Click File in the top menu then click open and then click external file. A window opens and at this point, you help each student browse to where they saved their pictures.

5: The pictures should show up on the window named noname1. Now, you click Boxes in the top menu and then Box count in the drop down menu.

6: Another window opens with the values of the dimension of the picture.

7: Now you can try it with different pictures of lungs from different animals and compare the values.

Activity 3

In this activity you will be creating Sierpinski's carpet using a 2cm x 2cm grid paper. You will make observations that compares the Sierpinski's carpet to the triangle.

Materials/Resources:

2cm x 2cm grid paper, rulers, markers

Directions:

1: Using the grid paper, draw a square with sides measuring 18 cm.

2: Divide the 18cm x 18cm square into nine smaller squares. Shade in the center square.

3: Divide each 6cm x 6cm square into nine smaller squares. Shade in the centers.

4: Divide each 2cm x 2cm square into nine smaller squares. Shade in the centers.

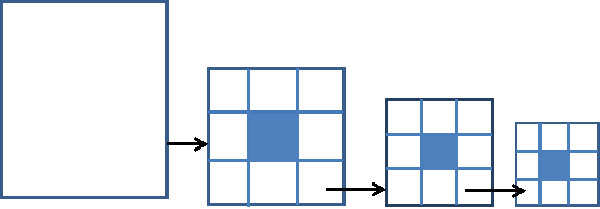
Answer the following questions:

1. Comparing this to the Sierpinski's triangle what do you notice?

2. Is there any similarities?

3. What about their dimensions? Are they the same?

Works Cited:

Bandt, Christoph, Peter Mörters, and Martina Zähle. Fractal geometry and stochastics IV . Basel: Birkhäuser, 2009. This book is acollection of articles about fractals in nature and new developments of fractals.

Barnsley, M. F.. Fractals everywhere . Boston: Academic Press, 1988. This book vividly explains all about Iterated Function Systems. I personally found this book easy to read and very enlightening.

Bassingthwaighte, James B., Larry S. Liebovitch, and Bruce J. West. Fractal physiology. New York: Published for the American Physiological Society by Oxford University Press, 1994. This book looks at mechanisms that generate fractals and they generate them. This book is also a bit of an advance read for me.

Kapit, Wynn, Robert I. Macey, and Esmail Meisami. The physiology coloring book. 2nd ed. San Francisco: Addison Wesley Longman, 2000. This book helps you understand the organ system by coloring. You are following a set of rules while coloring which makes it even more fun and understanding.

Lapidus, Michel L., and Machiel Frankenhuysen. Fractal geometry, complex dimensions and zeta functions geometry and spectra of fractal strings. New York: Springer, 2006. This book takes a look at fractal dimension and how to calculate them. This book will really benefit teachers that teach high school and college level students. It looks at some really cool and complex fractals and how to calculate their dimensions.

Mandelbrot, B. B.. The fractal geometry of nature . 1983 ed. New York, N.Y.: Freeman, 1983. This book a look at geometry of random fractals and also looks a wide range of pictures in nature containing fractals.

Peitgen, Heinz, H. Jürgens, and Dietmar Saupe. Chaos and fractals: new frontiers of science. New York: Springer-Verlag, 1992. This book looks at key concepts of fractals with lots of full color pictures. Teachers who teach other subjects other than math should of randombe able to pick this book, read and understand it.

Weyl, Hermann. Symmetry . Princeton: Princeton University Press, 1952. This book talks about the applications and principles of symmetry. It is a bit of an advanced read.

Appendix A: Useful Websites

http://www.mta.ca/~mctaylor/sci.fractals-faq/toc.html. This website has lots of questions and answers about fractals

http://cps-www.bu.edu/~trunfio/ogaf-programs.html. This link will help you understand fractal properties using the fractal software.

http://www.lerc.nasa.gov/Other_Groups/K-12/fracpage.html. This is a website created by NASA that answers the question "What are Fractals"

http://cml.rice.edu/~lanius/images/triangle.gif. You can find grid papers here for the Sierpinski's Triangle or Carpet activity courtesy of Cynthia Lanius

Appendix B: Links to beautiful examples of fractals you can explore

http://www.artvark.com/artvark/index/index.html

http://www.top.net/tim/fractal/fractal.html

http://snt.student.utwente.nl/~schol/gallery

Appendix C: Implementing NCTM Geometry Standards

Grades 6–8 Expectations: In grades 6–8 all students should–

- precisely describe, classify, and understand relationships among types of two- and three-dimensional objects using their defining properties;

- understand relationships among the angles, side lengths, perimeters, areas, and volumes of similar objects;

- create and critique inductive and deductive arguments concerning geometric ideas and relationships

- describe sizes, positions, and orientations of shapes under informal transformations such as flips, turns, slides, and scaling;

- examine the congruence, similarity, and line or rotational symmetry of objects using transformations.

- draw geometric objects with specified properties, such as side lengths or angle measures;

- use two-dimensional representations of three-dimensional objects to visualize and solve problems such as those involving surface area and volume;

- use visual tools such as networks to represent and solve problems;

- use geometric models to represent and explain numerical and algebraic relationships;

- recognize and apply geometric ideas and relationships in areas outside the mathematics classroom, such as art, science, and everyday life.