Activities
Activity One
The initial discussion will take place on the first day of the lesson, and will be preceded by some still photographs that I will put up on the Smartboard. The famous cover of the November 1990 issue of LIFE magazine is one picture I would like my students to see, and I will supplement it with a few others, readily available on the web. The discussion will begin with an open-ended question that will ask the students if and how AIDS has affected their lives or the lives of their families and friends. I hope to eventually guide the discussion toward deeper understanding of the early years of the epidemic, before they were born, and what it was like for people who lived and died then, showing images from that time as the discussion proceeds. I want to record key words and memorable text from what we hear that day, and have these translated to a handout for student reference.
Activity Two
I have been involved in several trials in the Bay Area, and I am affiliated with SCOPE, a research organization in San Francisco that is funded in part by several drug companies, and is housed at San Francisco General Hospital, and operates in conjunction with the AIDS ward of the hospital. There is a strong possibility that I could get one of the researchers I know to talk to my class about what it is like to work in that field, the science, the politics, the money, and how AIDS has changed so much in the last thirty year. There is also the possibility that I could take the class on a field trip to the hospital, so they can get at least one picture of how a medical research team works. That might be fine with a small class.
Activity Three
As is detailed in the content section of the unit, there are several places in the life cycle of the HIV virus where science has successfully 'inhibited' its propagation with antiretroviral drug therapies. There are entry inhibitors (EI), reverse transcriptase inhibitors (RTI), integrase inhibitors (II), and protease inhibitors (PI), and then several different types of these. I would like to use the classic jigsaw strategy here, breaking the students into groups of three, four, or five, depending on class size, for the purpose of presenting to the class their particular piece of the puzzle which represents one step in the chain of events which inhibit the virus.
The day before the presentations, we will watch several animations on the Smartboard, the best one I found being 'Retrovirus Replication 3D Animation' on You Tube, and images from several other sites, some of which are listed in the last section of this paper. The students will have to do a small amount of research, with notes of some kind, to assist them in their presentation. They will be expected to answer questions posed by the other groups, and the interaction should strengthen their understanding, since each of the inhibitors have the same purpose, but accomplish it in different ways.
Activity Four
Jesse Gelsinger, a high school student, died from the result of a clinical trial involving gene therapy in 1999, at the age of eighteen. He suffered from a genetic liver disease, and was injected with an adenoviral vector carrying a corrective gene. He suffered a massive immune response triggered by the vector, and died of multiple organ failure and brain death several days later. His death represented a major setback for research in that field for several years afterwards. He is the first known death attributed to a gene therapy trial.
There will be a class discussion about what gene therapy is, and how it has also proven to be beneficial, especially for people who suffer from genetic diseases such as X-linked adrenoleukodystrophy, Metachromatic Leukodystrophy, and Wiskott-Aldrich syndrome, all mentioned in a previous section of this paper. This will be accompanied by still pictures, and several animations.
A debate on gene therapy will begin the following day, with students either signing up as pro or con, or as assigned by the instructor, or a combination of both. All students will be required to write and submit a short research paper about Jesse, and include other information they feel supports their pro or con stand on gene therapy trials. It will hopefully be a lively one.
Activity Five
This is a simple probability exercise using theoretical statistics about HIV infection. It can be done in class using a cooperative learning model with heterogeneous groups of students.
The HIV antibody test has been around since late 1984, and is now a relatively simple test, and results are back in minutes. It was a blood test originally, and results took up to two weeks to get back. The results show either a positive antibody response or a negative antibody response. However, it was possible, and it still is, to get either a false positive or a false negative result, although this is not likely.
Different data tables can be set up on a handout and distributed to the groups of students to work on in class, and compare, share, and explain their results. A population study is given, along with numbers of HIV+ and HIV- people, and positive and negative results for each group. An example table might look like this:
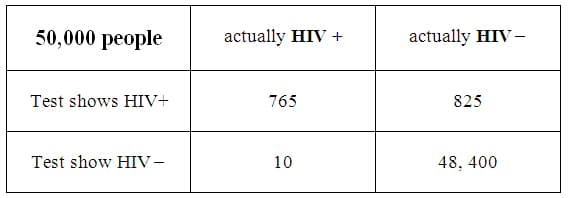
As can be seen from the table all four categories total to 50,000, and the students would then be asked to analyze the data given several prompts. These could have to do with the percentage of HIV+ people in the general population, the likelihood of getting false positives or false negatives, and the margin of error that can occur with either situation. As mentioned above, it would be a good idea to use several different data sets. A calculator may be used here, and each group will share its calculations and results with the class.
Activity Six
This is a more complex probability activity that involves viral mutation rates, and the ability of HIV to 'break out' of an established antiviral regimen, and restart its infection.
It has been established that the error rate of reverse transcriptase is very high when compared to cellular DNA, so there is always a chance of virus being released whose genome is sufficiently altered such that it becomes resistant to one or more of the antiviral drugs. Because there are four major events in the virus life cycle at which these drugs can stop the virus, and several different drugs which use different mechanisms to do that, this sets up a lot of possibilities for estimating the probability of a break out virus. This activity could be used in a different way than presented here. For instance, break out probabilities might be considered when only one inhibitor is being used rather than two, three, or all four, to simplify the activity. The following is the standard version.
Step One: determining viral load
Let's say we are a group of doctors and nurses working with an HIV patient who has never had any antiviral therapy. Our patient is producing 500,000,000 viruses a day that enter the blood, which would be typical of such a patient. Let's say this person is male, and the average male body contains 5 liters of blood. 'Viral load' is a measure of the amount of virus in a particular individual, and is defined as the number of viruses per milliliter of blood. Under these circumstances, what would the viral load be in our patient? Please give an answer and a short explanation of how you got it.
Step Two: Using entry inhibitors
Let's consider those viruses which are in the single milliliter of blood in the immediate vicinity of a group of cells. We will consider these to be viruses that can potentially enter some cell, which is the first step in infection. There are two different ways for the virus to enter and they are the two receptors on the cell surface called CCR5 and CXCR4 . Let's say that our patient is taking an antiviral that makes it difficult for the virus to interact with the CCR5 receptors, and 80% of the virus that come in contact with the cell cannot fuse with the cell using this receptor. What fraction (and percent) of the virus in this single milliliter of blood that could possibly enter the cell? How many virons does this fraction represent?
Let's now assume that the remaining virus which can use the CCR5 must also use the other receptor CXCR4 to finally enter the cell. Now let's now give our patient both antivirals, one for each receptor. The antiviral for the CXCR4 receptor will be effective in blocking about 40% of the viruses that were able to successful interact with the CCR5 receptors. Now what fraction and what percent of the virus that has successfully used both receptors in this single milliliter of blood will gain entry into a cell in this patient? How many viruses in this millimeter of blood will gain access to a cell?
Step Three: using reverse transcriptase inhibitors
There are two different types of reverse transcriptase inhibitors, nucleoside reverse transcriptase inhibitors (NRTI), and non-nucleoside reverse transcriptase inhibitors (NNRTI). NRTIs are competitive inhibitors which act as dummy nucleotides. They get inserted into the copy of the viral genome, and contain a defect which halts the copy process. They are therefore referred to as competitive inhibitors, since they compete with one of the four bases (Adenine, Thymine, Cytosine, and Guanine) in the growing chain of cDNA. NNRTIs inhibit the process externally by distorting positions of key elements in the process, and are thus referred to as non-competitive inhibitors. RNA contains a base called Uracil, which functions pretty much the same as Thymine.
So, let's give our patient one of the NRTIs. And let's further say that in order for our patient's virus to become resistant to this NRTI, it must be missing a sequence of 10 identical nucleotides that normally occur right in a row in one location on our patient's virus, TTTTTTTTTT, for example. The average HIV genome is 1,000 base pair long, and on average one error is made for every 1000 base pair, thereby creating a mutation, and making the cDNA molecule unrecognizable to the NRTI, and therefore resistant to it. What is the probability that this error will occur along this particular sequence of ten bases? Considering how many viruses were capable of entering cells, combined with the probability of NRTI resistance, how many viruses in our milliliter of blood still have the potential to complete the viral life cycle?
If we also give our patient an NNRTI, this will also further inhibit viral replication. Let's say that NNTRIs are successful at distorting 20% of the mutated viruses which are resistant to the NTRIs. What is the percent chance that a strain will be produced that will be resistant to both the NTRIs and the NNTRI from the pool of virus that entered a cell? How many virus are still a potential threat to our patient?
Step Four: using integrase inhibitors
The remaining viruses which have entered a cell, and gotten past the RTIs, now move into the nucleus to be integrated into our patient's DNA. We can treat our patient with an integrase inhibitor, which will prevent this from happening, at least in most cases. With that in mind, let's say that in order for our patient's virus to override the inhibitor, it must be missing a sequence of AGCT that occurs randomly at some place in the viral genome that the inhibitor recognizes. On average, and to the nearest whole number, how many times would we expect this sequence to repeat on the 1000 base pair long HIV genome? The number of viruses that resisted the integrase inhibitor can be calculated by dividing the number of viruses that entered the nucleus by the number you just found. How many viruses were able to integrate themselves into our patient's DNA?
Step Five: using protease inhibitors
The final opportunity to interfere with viral production is when the replicated viral genomes need to be segmented for the purpose of being packaged and exiting the cell. This is where the protease inhibitors come into play. For a virus to become resistant to and bypass the protease inhibitor, it must be missing a certain sequence in the location where the inhibitor binds, that occurs, on average, in one out of every twelve of the remaining viruses. These are the totally resistant viruses that will be packaged and exit the cell to possibly infect other cells in our patient. Of the 500,000,000 viruses we started with, how many remain that will exit the cell into our patient's body as totally resistant virus?
What might you speculate about this person's short and long term survival, considering your final result?
Activity 7
Finally, there will be a test on probability in Algebra II, and several questions about HIV will appear there, integrating math and science, as was my intention from the very beginning.

Comments: