Content
It is imperative that students develop significantly more than just a level of comfort with understanding fractions. Students need multiple exposures with solving real life problems with fractions in order to gain a deeper understanding of the various imbedded concepts.
I will focus on the following topics in this unit.
- A fraction is a number.
- Fractions can all fit into a coherent system on the number line.
- Fractions can be ordered according to size.
- Fractions can be added.
- That each rational number has many names.
I will also devote some attention as time allows to the more advanced topic
6. That numbers are ratios.However, this idea in implicit form will form a constant refrain throughout the unit.
The second topic, that fractions can form a coherent system that can be visualized with the help of the number line, will be one of the main tools for developing all the other topics. We will also make some use of area models, especially brownie-pan type models. I hope to look at the potential strengths and weaknesses of both. Activities suggested will align with the expectations of the Common Core State Standards.
Fractions show up in many aspects of daily living and in our careers. The variations of problem types can be classified as levels or dimensions in which we teach or see them appear in our lives.
- One dimensional - linear model, whether they appear horizontal or vertical; which include but are not limited to: fraction strips, rulers, foot plates on sewing machines, thermometers, liquid droppers, or the calibration on an object for determining a particular aperture; and most commonly, the number line.
- Two dimensional- Area models; used with primary students addressing the part- whole concept. Commonly used area models include: geometric shapes, pie plates/, pizza models, brownie pans, and attribute blocks. Note that pizza and pie plate models, or any circular model, is essentially a linear model, but with more complicated geometry. The brownie pan or "cornbread" model allows one to take advantage of two-dimensional geometry and area to work with fractions.
- Three dimensional – using measuring cups to find a capacity or volume of a liquid or solid.
- Ratios- Fractions which describe either a part-part or part-whole relationship. In fact, all numbers are ratios, and ratios are numbers. But our curricula do not make this clear. Example: "The idea of, the number of children using the swings on the playground compared to those children using the slides. Or those children using the swings compared to the total number of children on the playground. The discussion of ratios as fractions is situated within the 6 th grade Common Core." 4
Each of these concepts of fractions has value and is important within a particular pedagogical development and context. This unit will not explore three dimensional models for fractions.
Fractions are Numbers
I introduce new subject matter and abstract ideas through a concrete format. "Understanding fractions proceeds best in a balance of three stages: using manipulatives for concrete representations; using pictorials as semi-concrete representations; and, finally using numbers to represent abstract relationships involving procedures and operations." "The process of moving from concrete representations to abstract, is a good model to begin with and it is almost essential when doing sharing tasks." 5 ? The goal of using a number line to model fractions is to help students realize that, just as for whole numbers, each fraction corresponds to a specific point on a number line. Students tend not to view fractions as numbers with specified points on a line, but instead as a portion of a whole. The Common Core suggests approaching fractions by starting with unit fractions. Consider fourths as an example. First, one needs to understand that 1/4 is one of four equal parts of a given unit. It takes 4 copies of ¼ to make the whole unit. Then ¾ is defined as 3 copies of ¼:
¾ = ¼ + ¼ + ¼ = 3×¼. Display.
I will illustrate these ideas on a number line. When we start working with a number line, I want to make sure that my students understand that a number on the number line is telling how far a point is from the 0 or endpoint, in relation to the unit length. So, on a number line, the unit fraction1/4 labels the point at distance 1/4 of the unit distance from the origin (0). There are four exact iterations of it within the distance from zero to one. By placing 1/4 on a number line and ½ on a number line it becomes visually obvious that these fractions are different distances from the origin. With 1/4 one will need four exact copies of the 1/4 length to complete the unit interval, whereas, for ½ only two copies will be needed to complete the unit. See Figure 1. The denominator indicates the number of iterations needed to complete a unit. I plan to have students label the unit fractions and their multiples (general fractions) on a number line. We will look at the multiples of 1/d for several denominators d, so that students can see both the compelling structure of the system of fractions with a fixed denominator, and can also see that, as the denominator gets larger, its multiples become more finely spaced, corresponding to the fact that a unit fraction gets smaller as its denominator gets larger. It is an important pattern for me to establish early on in my teaching that the larger d, the smaller 1/d: it takes more copies to make 1.
Figure 1
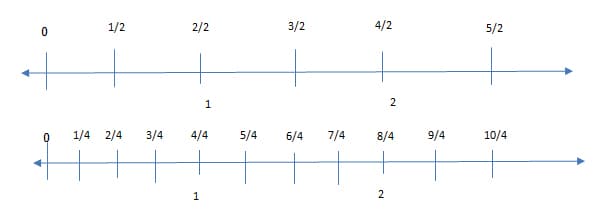
When students are asked to form a conjecture about which size fraction is greater, it is a common misconception for students to believe "the larger the denominator, the larger the size of the fraction." It is not uncommon for elementary students when asked, "Would you rather have one eighth or one fifth of a pizza?" to respond, "one eighth." Using number lines in my classroom opened the door to bring home the concept that the larger the denominator the more iterations or copies needed to make the unit, and therefore, the smaller the unit fraction. In the situation of ¼, the distance from the origin to ¼ is smaller than the distance between the origin and ½. With repeated learning experiences, students come to understand that the larger the denominator the smaller the unit. The reverse is also true, in that the smaller the denominator the larger the unit fraction. I have found that my students need repeated opportunities to divide same size units into any given number of parts in order for this relationship to be understood. Students should be able to express, for example, that subdividing a unit into eighths creates smaller fractional parts than when subdividing the same size unit into fifths. Lessons will be purposefully designed to bring out how the size of a fraction is affected by the digit in the denominator. With whole numbers students are accustomed to: the larger the number the more of said items one has. With fractions however, the larger the denominator the more finely subdivided the unit interval will be. The number line is a learning tool which provides a concrete model for students to easily see the size of unit fractions.
With the number line, students can also compare where one half and one fourth lie in comparison to each other and notice that one fourth is half the size of one half. Other conversations that are generated when placing fractions on a number line include: their distances from the origin, how many iterations, including the original, are needed for a complete unit to be created, numbers having multiple names and the vocabulary of: improper fraction, mixed numbers and equivalent fractions. These concepts no longer have to be taught in isolation. In comparison, the area model provides a clear visual as to the size of the parts compared to the size of the total unit. The area model does not however provide the comprehensive picture that the number line does, of all fractions in order, with larger ones farther from 0, and smaller ones closer.. Fractions can all fit into a coherent system on the number line.
As a teacher, I find it necessary to reflect on and readjust my lessons to meet the needs of the students. Reflecting upon my teaching of fractions, I historically asked students to label fractions on a number line focusing between zero and one. Recently, I realized that I needed to extend the work beyond the unit interval. When my lessons confined the learning and focused only between zero and one, students did not intrinsically transfer the idea that fractions existed also at and between other whole numbers. I have learned that students need to be exposed to as many view points as possible. Now when I introduce a new concept, I build in discussing all of what it is, as well as, sharing also what it is not. I use a tool called a Word Wizard to provide students space to record ideas associated with new vocabulary and concepts. See attachment A. I have seen an increased understanding of fraction concepts in my students since labeling fractions on a number line beyond 1 and using the word wizard. In creating the number lines as in Figure 1, I have my students count below the number line by halves: 0, ½, 1, 1 ½, 2….. Above the number line we indicate the number of copies of the unit fraction: one half, two halves, three halves….. Without having a formal lesson on fraction equivalence, students will see that each number has more than one name. For example, we can rewrite one whole as two halves or four fourths. While creating number lines I ask my students to look for patterns. By looking for patterns students will notice that in order to rewrite, two, as a fraction the numerator has to be twice the denominator. When we extend the number line, they see the pattern continues. At three wholes the numerator will be three times the denominator, then four times at four. Having students look for patterns is what the Common Core Practices refers to as Look for and Make use of Structure, Standard 7.
Throughout my career, I have generally observed the brownie pan/area model being used as the primary tool to teach fractions. Area models in the sense of planar figures can involve many shapes. Pie/pizza models are attractive and use familiar objects, but the brownie pan model has so much more flexibility. Especially, it can be used to deal with repeated subdivision, reconstitution and renaming, which are central to understanding fractions. I tended to rely heavily on this model to bring out the concepts of parts and wholes. My colleagues and I repeatedly enforced ¾ as three out of four and ritualistically asked students: What do the three and the four stand for? Eventually the classes in chorus responded: "the three represents the parts and the four is the number of parts in the whole."
But did they really understand what the whole refers to? Have the students grasped the concept of being less than one? Did they realize there are fractions that are greater than one? I wonder; when students come to fifth grade, I ask: Can anyone name a number between zero and one. Typically, they stare at me as if I had grown a third eye. I believe that the rigid or sole use of area models have missed their mark in being able to clear some of the cloudiness that seems to hover over curriculum units on fractions. The Common Core Standards suggests that it would have been better, for my colleagues and I, to emphasize the relationship of the parts to the whole: it takes 4 ¼ s to make the whole. The unit fraction is the symbolic expression of that relationship. The first thing to do is to set up situations that help students transition to thinking about numbers as ratios, meaning, expressing quantity relationships, rather than as counts. The unit fraction approach can promote this.
With the fraction ¾, students view the three and four as whole parts, there are three whole slices of pizza out of four whole slices from the original pie. A slice of pizza is considered a whole piece to a child. The only way they would not consider it a whole is if the slice was cut in half or mangled in some way. Then and only then would I hear a child say, "I only have a half, or part of it." Three and four, in ¾, continue to maintain a skewed sense of wholeness, as students are most familiar in elementary school counting by whole numbers. One needs to change the focus, from the number of parts to the size of the parts relative to the whole. The ¼ is the relation between the part and the whole. The ¼ piece is a unit unto itself. Its 1/4 –thness lies in its relationship to the whole pizza. This is why fractions are hard – they involve rethinking what a number means. My job in teaching fractions is, first to understand this way of thinking about numbers, and second, to help students to adopt it.
The use of pies, brownie pans and pizza's clearly aid students in understanding the idea that fractions have two parts and that the parts are related. The idea that there is a part that identifies how much is shaded or is missing; the numerator, and the denominator, the number of total parts, has hit home. The idea that ¾ is one number and that number is among other numbers in a linear order, having numbers both before and after it, has not sunk in. The number line provides a linear model where students can, in an addition problem, see the number "grow."
Also, fractions represented in bars of appropriate lengths, can be laid end to end on a number line to find the sum. I have seen both fraction bars and Cuisenaire Rods used to demonstrate the linear model. Initially, students will perform this activity with fixed denominators and gradually progress to more sophisticated situations and mixed denominators.
Linear models resemble the process where primary students create trains to represent the addends in addition problems and calculate the sum. Using a familiar process will import prior knowledge to new concepts. The idea of connecting prior knowledge to new concepts was studied and coined: the Constructivism Theory. According to the Theory of Constructivism, all people, construct or give meaning to things they perceive or think about. The tools you use to build understanding are your existing ideas and knowledge. Your materials might be things you see, hear, or touch or they might be your own thoughts and ideas. The effort required to construct knowledge and understanding is reflective thought. Through reflective thought, people connect existing ideas to new information and thus modify their existing schemas to incorporate new ideas. Finding ways to build connections from prior knowledge to new concepts is important.
Comparing and Ordering Fractions
Once the concepts of units and unit fractions have been acquired, students should be ready to transition into comparing and ordering fraction. In prior sections, the number line has been suggested to be used to provide a concrete tool to assist students in being able to visualize fractions. They have been counting iterations of fractions with a fixed denominator, one half, two halves, three halves… Within this next set of concepts, comparing and ordering fractions, students will be using a number line to determine the relationship between any unit fraction or general fraction (a fraction greater than one unit fraction), and benchmarks of zero, one half or one whole. I will have my class work in pairs placing a given fraction on the number line with labels: zero, half and one whole. I will circulate to make notes of how students are determining if the fraction is closer to zero, half or a whole. These notes may include: which students are struggling to correctly place the fraction on the number line, which students are successful, a student's remarks that expressed a concept embedded in the day's lesson…
Another activity I plan is to have students sort a set of fraction cards into piles of closer/equal to zero, closer/equal to half, or closer/equal to a whole. In both activities, I will ask student pairs to share their results with the class. Any misconceptions should be discussed and students should feel comfortable to express questions they may have concerning their own cards' placement, or to challenge their peers, if they disagree with a placement of a card. Learning outcomes from this activity should include students being able to conclude:
- Fractions with numerators equal to half the denominator are equal to one half.
- Fractions with numerators less than half the denominator lie between zero and one half.
- Fractions with numerators greater than half the denominator lie between one half and one whole.
- Numerators larger than the denominator are greater than one whole.
The ability to place fractions on a number line and determine whether they are closer to zero, half or one whole is situated in the fourth grade Common Core Curriculum. 4.NF.A See Standards
Students who struggle with the activity should continue to use concrete objects, area models or previously constructed number lines. The area model will easily allow students to visually identify if the whole is barely filled in, partially filled in or almost completely filled in. The idea behind this activity is to begin having students think about estimating with fractions and solidify the concept of the relationship that exists between the numerator and the denominator. Estimation is one of the most effective ways to build understanding and procedural fluency with fractions. A mental image or awareness of the size of a fraction helps students assess their answers for reasonableness. 6
I plan to keep a large number line in the classroom. Each day we will add a new fraction to the line. A clothes line and clothes pins works nicely. From a set of fraction cards, each day I will select a student or students to place a new card on the number line. The class can agree or disagree with the placement of the card, and he/she will need to provide a rationale. It is during these conversations that I can gather some formative assessments by listening for ways in which students are thinking about the fractions. I listen for students to mention whether the new fraction should be placed before or after previously placed fractions. Or whether this fraction has a larger denominator and would have more closely spaced units and should go in the same place as a fraction that is already there. This will also give me a chance to reinforce the understanding that the numbers are expressing distance from the origin. It may also be valuable to look at the unit fraction represented by the denominator, and see how many copies of that go into making today's fraction. Our students are fortunate to have access to ipads. They use the following apps to practice ordering fractions on number lines: Fractions and FracLine. I can assess their individual understanding of ordering fractions when monitoring their ipad time. The student's ability to justify and defend the reasonableness of their solution is what the Common Core Mathematical Practices refer to as, Look for and Make Use of Structure. Arguably, one might also say it is, Attend to Precision, depending on how specific the conversation becomes. The conversations themselves point to Mathematical Practice 3, Construct Viable Arguments; the whole reason for the title of this unit. I want students to understand fractions to the point in which they can construct an argument that will defend that the placement of the fraction on the number line is reasonable. Through this process of adding fractions to the number line, students have now moved from comparing fractions to benchmarks to comparing and ordering fractions with differing denominators. Lessons should support and offer connections to other algorithms that aid in comparing and ordering fractions. These lessons should include renaming each fraction with a common denominator by use of creating number lines that are subdivided by multiples of the unit fraction with denominator equal to the product of the original two fractions. Fractions with large denominators will most likely become cumbersome to students and in comparing fractions the area model may be more realistic for elementary students to use. Through use of the area models students will see how to rename fractions with a common denominator and should eventually move to more abstract ideas and an algorithm.
Students who have a strong concept of fractions may benefit from moving to a more efficient method of comparing fractions, by using the Cross Multiplication Method. This method has its mathematical foundation deeply rooted in the area model. The Cross Multiplication process for comparing two fractions is as follows: one multiplies the denominator of one fraction with the numerator of the other fraction, and records the number above the fraction, like a superscript. For example: when comparing 2/5 and 1/3. The denominator, three, in 1/3, is multiplied by the numerator two, in 2/5, for an answer of six. A superscript of 6 is placed above the two in 2/5. Then, the denominator five, in 2/5 is multiplied by the numerator one, in 1/3 for an answer of five, and a superscript of five is written above 1/3. A comparison is now done of the two numbers that have been superscripted: six and five. Since six is greater than five and is associated with 2/5, we conclude 2/5 is greater than 1/3. See Figure 2. When students write real world problems using numbers and symbols such as greater than, less than or equal to, they are performing Common Core Practice 2: Reason Abstractly and Quantitatively.
Figure 2
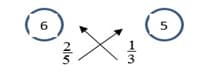
It has been suggested that the Cross Multiplication Method is procedural in nature and does not provide students with a conceptual understanding for how or why it works. If students are comfortable with renaming, then this provides a simple explanation. We know by the principle of renaming that
1/3 = 1×5/3×5 = 5/15, and 2/5 = 2×3/5×3 = 6/15. Since 5/15 < 6/15, then 1/3 < 2/5.
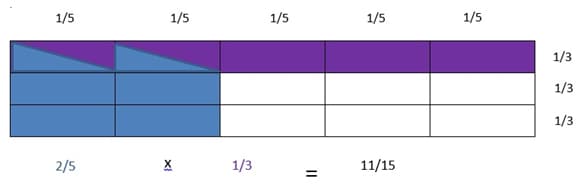
The renaming process can be represented visually using the area model. The six and the five, in this example, represents the number of parts that would be shaded, had an area model been created to represent both fractions as parts of the same whole. See Figure 3... In that figure, we see that when we create an area model with vertical partitions that shows 2/5 horizontal lines that represents1/3; we see that fifths have been subdivided into thirds and thirds into fifths creating fifteen equal parts, each of which is therefore 1/15 of the whole. 2/5 is now represented as 6/15 of the model and 1/3 is represented as 5/15 of the model. So 2/5 = 6/15 > 5/15 = 1/3. The superscripted numbers are the numerators created when finding the common multiple for five and three. The Cross Multiplication process is simply a more efficient way of determining the size of the fraction rather than creating a drawing with the area model. Cross multiplication is a procedure that should be taught only as a more efficient means of computation, after students have learned how its simplicity came about from repeated use of the area model
Figure 3
A second method for students to compare and order fractions is to convert the fraction to a decimal. Some students more readily compare decimals than fractions, especially those written with only two digits, as they associate this and the nomenclature for money. Something they have had multiple prior experiences in previous grade levels. This method lends well to connecting the concept of fractions and division. Student need to be exposed to the concept that ¾ means three divided by four. When they perform the function, it creates a decimal. Students can use calculators to alleviate the tediousness of changing fractions into decimals. Changing a fraction to a decimal can create a lengthy decimal. Thus, one needs to agree to understand that in changing a fraction to a decimal in order to compare them, will be but an estimation of the fraction, since we tend to round them when they become lengthy.
Adding and Subtracting Fractions
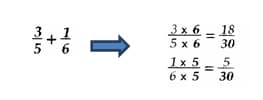
According to Fifth Grade Common Core State Standards 5.NF.A.1 and 5.NF.B.4, students are to add and subtract fractions and mixed numbers by replacing a given fraction with equivalent fractions and they should also use visual fraction models to assess the reasonableness of answers. If students over the course of their studies of fractions, have been engaged in creating area models and creating multiple number lines; if they have been engaging in rich conversations about unit fractions and units; if they have been comparing fractions and able to debate whether a given fraction is greater than, less than, or equal to another, students should receive these new concepts in fifth grade with ease. When student compared fractions by creating area models, this in fact created equivalent fractions for each of the given fractions. The students were then able to compare which fraction was larger or smaller, since each fraction was written with like denominators. With adding and subtracting fractions, students can continue to use area models to create equivalent fractions, as they did in fourth grade, but instead of compare, now add or subtract the newly created fractions to find a sum or difference. For example: ½ + 1/3 = 5/6.
Figure 4

Over time, with repeated use of models students will come to understand that any set of fractions can be renamed as fractions with a common denominator. In order to move students toward using a more formal algorithm, they will also need to understand that multiplying both the numerator and the denominator by the same number creates an equivalent fraction. As they physically performed subdividing the area by the denominators, students will see that they have created an equivalent fraction of sixths. Students who may display perceptual problems would benefit from using ruled paper turned vertically to keep the numbers orderly when initially performing the algorithm for adding and subtracting fractions. This will be especially helpful also when adding and subtracting mixed numbers. It is also important to note, curriculum commonly display fraction problems horizontally, and I encourage students to re-write the problems vertically. Students may want/need to continue to use area models as proof that they reconstituted the fractions correctly. Example:
Along with adding and subtracting fractions, students in fifth grade are expected to evaluate the reasonableness of an addition or subtraction fraction problem. The concept of determining reasonableness of a fraction being closer to zero, closer to half or closer to one whole was introduced in fourth grade and is reappearing in fifth, but within a new context. Students are expected to bring forth previously learned methods of estimating fractions and adding fractions. They can continue to rely on area models and number lines. The goal however is that the prior learning has led students to being able to estimate each fraction. Given an addition or subtraction problem and its solution, students are to argue, through use of mathematical reasoning's, if the sum or difference is a viable solution. For example: students will be asked to examine an equations like: 6/8 + 2/5 = 2. In the example, can two be a reasonable solution? A student may conclude that 6/8 is less than one and 2/5 is less than one, so the sum must be less than two. Students generally enjoy a game with some competitiveness. Using dominos, I will have students chose six dominos with at least two pips. Also I will make 12 cards labeled with either a one or a two. Assign one student as the number one and the other as the two. Students randomly select one of their dominos and one of the cards to create a joint addition number sentence. Example: one domino may have four pips and six pips. A student could assign this domino the fraction 4/6 or 6/4. The second domino may have eight and twelve pips. The student may decide to name this domino 8/12 or 12/8. Once they have decided which fraction they want their domino to represent, they record it as an addition problem. One of the 12 cards is randomly drawn as the estimated sum to the addition problem. If the number sentence is reasonable and the solution was one, the student assigned to number one collects and keeps the dominos from that round. Had the number two been the selected solution card but the solution is one, no one collects the domino, and a pile grows until the next opportunity to win them. The student with the most dominoes after six rounds wins. For assessment purposes I have students record the results of each round on a scoring sheet. For students who struggle, I return to estimating single fractions and then link the idea of adding or subtracting the parts creating their own sum or difference and comparing that solution to the given solution.
Rational Numbers
Within the course of creating a number line with multiple whole units, students will naturally see fractions extending beyond one whole unit. These units will be labeled with what we familiarly refer to as improper fractions. I do not necessarily refer to them as improper fractions, mixed numbers or equivalent fractions initially. However, during lessons where students are counting; one half, two halves, three halves, I want to challenge them to explain why they are able to rename four halves as two or four eighths as one half. Struggling students will need familiar, concrete, objects to bond with this concept. See activity one. Through repeated use of counting by various fraction on the number line, students will also reach the conclusion that each rational number has many names. It is no longer necessary to teach one lesson on improper fractions, one on mixed numbers and then create an algorithm to help students go from one to the other, then back again. These ideas and concepts can be developed simultaneously as students see the number two is also eight fourths, and that nine and a half, halves is also four whole and one half. This is what the Common Core Practices refer to as: Look For and Make Use of Structure. I want my students to be able to see and understand the flexibility of numbers.
Fractions as Ratios
According to the Free Merriam-Webster Dictionary, a ratio is the relationship that exists between the size, number or amount of two things. It is impossible to talk about a number without relating it to something. Numbers are merely adjectives that describe nouns. Specifically, they tell how much there is of some noun, relative to a given unit. One cannot physically demonstrate what five is without representing it with a unit. If you ask your class to show you five, students may show five fingers or five pencils, but they are not able to show five. We infer five, when we see five units of fingers or pencils. They in themselves are not five, but there are five of them. Five describes the number of pencils or fingers, a unit, chosen to represent, in this case, the number five. It is important that students develop the habit of identifying the unit to which any given number, and especially a fraction, refers. I plan always to require students to write or express their answers with the unit identified. This requirement will assist students in becoming consciously aware of the concept that numbers are used to represent real life units, and without knowing what the unit is, the number has no significance. I cannot clearly understand what five is until I know what unit it is associated to. Hand me five pennies and I know I have five cents. Hand me five one dollar bills and that is a very different amount! If you tell me, you are sending me to the store with five, I don't know what I will be able to purchase. Fractions work the same way. We must know the unit to which they refer to understand what we actually have. Children learn to count one dog, two dogs, three dogs…. Once understanding, for example for fifths, 1/5 is a unit, just as a dog is a unit, in the example; students will know, when counting by fifths that the numerator will increase, but not the denominator. Grounding this concept of counting on the number line by fractional iterations, students will be able to add, subtract, and multiply fractions with greater ease. A simple subtraction problem might read: A pie is cut into 8 pieces. Ann eats 5/8 of the pie. How many pieces of pie are left? Knowing that Ann ate 5 pieces, students could count on 6/8, 7/8, 8/8 and determine there are three pieces left.
Oddly enough, we use fractional units routinely in our daily lives, but may not have thought of them as such. I try to build connections and link student's knowledge of what they already know about units to the newer concept of unit fractions which I am trying to instill. The idea is to bridge old ideas to new concepts to form stronger more inclusive definition of a concept. Included in the appendix is a set of cards that can be played by a single player, in pairs, small groups or teacher against the class. The activity is meant to assist in building connections between prior knowledge and new Common Core State Standard's concepts of how the unit fraction relates to the unit. To elicit the prior knowledge and make connections to unit fractions, I have asked: "What fractional part is a quart to a gallon?" The answer is, ¼. Similarly, you can invert the question and center it around the fraction, by stating: What is ¼ of a gallon? The answer is one quart. I will also point out unit fractions when discussing time, money, length and other measurement concepts that my students know. For example: What fraction of one hour is one minute? What is one tenth of a dollar? What is 1/7 of a week? The answers are 1/60, a dime, and a day, respectively. The units in each of these scenarios are the dollar, a gallon, and the week. The dime, quarter and day are referred to as the unit fraction. A dime is 1/10th of a dollar. A unit fraction is mathematically written 1/d, meaning: 1/d of some unit, such that it takes d of them to make the original unit, d times the numerator make a complete unit. In this situation, ten replications of the unit fraction (dimes) are needed to create the dollar. Ten dimes equals the equivalent unit of 1 dollar. The quart is ¼ of one gallon; when you have 4 times the one quart, you have a unit known as one gallon. These scenarios allow one to see the direct correlation between multiplication and division. In one scenario you are asked to find how many parts are in the unit, division. In the inverse question one is finding how many times of the unit fraction is needed to create the unit. Each of the unit fractions can stand independently. They define new units: a quart, a dime or a day. One can purchase a quart of milk. If you purchase four one quart containers the amount of milk you have is equal to one gallon of milk, even though you do not physically have a one gallon container of milk, you do have that amount of milk. There is an inherent relationship between the quart and the gallon, between minutes and hours, and days and weeks. The smaller item in the pair is the unit fraction when the larger item in the pair is designated as the unit. The relationship that exists between the examples is undeniable. It is this relationship that connects the ideas of unit fraction and units to our everyday lives. It also confirms numbers are ratios in that some quantity is being compared to the unit being by which it is being measured.

Comments: