Introduction
Teaching mathematics in High School involves multiple challenges. Among these are the lack of students’ interest in learning the subject, the generalized notion that mathematics is a complex subject, and the belief that what students learn has minimum or null real-life applications. In my teaching experience, teaching word problems is an excellent way to address these challenges. Although students are not inclined to solve these problems and prefer simple symbolic ones, word problems have two advantages. First, they allow practicing reading comprehension, and second, they open the possibility of solving real-life, engaging, and meaningful real-life problems for the students.
Notably, when solving word problems, the main task is translating the given text into equations (usually just one equation for simple problems) and then solving using algebraic rules. However, even if the first step is successful, students may only be able to answer the problems if they can do the algebraic work on solving the resulting equation. Among all kinds of equations, divisions of fractions cause trouble for my students. Furthermore, research shows that word problems involving fraction division are particularly challenging. For instance, Koichu et al. showed difficulties with the conceptualization of divisions of fractions by investigating the ways of thinking of mathematics teachers (Koichu 2013). The authors showed that problems are successfully solved when the given fractions are perceived as operands instead of reading the problems as a division of divisions, thus opening a path for successful teaching of this topic. These results support earlier findings by Ma, where participants were asked to calculate 1 ¾ ÷ ½. Notably, almost half of the participants confounded dividing by ½ with dividing by 2 (Ma 1999). Because of this result, Ma highlighted the need to replace the ‘‘uncomfortable’’ division (e.g., a fraction by a fraction) with a ‘‘comfortable’’ division (e.g., a fraction by a whole number).
Similar to these results, I see my Algebra II students confident when solving problems of divisions by whole numbers, while they are not when given problems of solving divisions of fractions. In the latter case, many accept that “new rules” apply for these kinds of problems, which are not clear but, if followed, can produce a correct, although not transparent, answer. An example is when I asked my students to solve the division of 4 by 2, and everyone answered 2. That is, dividing 4 into groups of 2 produced parts of size 2. Next, when asked to solve the division of 4 by ½, many recurred to the symbolic solution of 4 ÷ ½ = 4 * 2/1 = 8, without having a clear idea of what the 8 represented. In this sense, using models like the number line or the rectangle model promoted by the Singapore Ministry of Education can be very helpful (Singapore Ministry of Education 2003). When asked the problem: 4 ÷ ½, this translates to dividing 4 wholes into groups of ½. One good way, which works for divisions by whole numbers and division by fractions, is to think about dividing a by b by asking, “How many copies of b are in a?”.
This definition of division can be illustrated well using the number line and rectangular models in what is known as the “measurement model” of division. For example, for the above problem, using the rectangular model to solve 4 ÷ ½, we have the following representation in Figure 1.
Or:
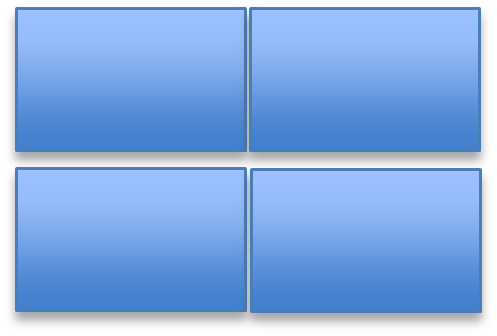
4 wholes:
Divided by ½:

Gives 8 copies of ½ size
Figure 1. The rectangular model represents the division of 4 by ½.
The same procedure works for the problem, 1 ¾ ÷ ½, that is, how many copies of ½ are in 1 ¾, but we will illustrate its solution using the number line as shown in Figure 2.

Figure 2. Number lines representing the division of 1 ¾ by ½
In the first number line, the red segment represents 1¾. Next, we have a second number line, marked in intervals of length ½, ½’s, to determine how many copies of ½ are in 1¾. We can see from the blue line that ½ fits 3 and ½ times, which is the answer to our problem.
These models are visual representations of the division by fractions. They are very much needed to provide a rationale for students of the arithmetic operation, an argument supported by Li, who says that learning mathematics needs to go beyond rote memorization of procedures since its meaning requires explanation through connections with other mathematical knowledge, various representations, and/or real-world contexts like the above-described models (Li 2008).
This unit uses three collections of word problems to teach Algebra II students how to solve real-life applications using one simple algebraic equation whose solution involves the division of fractions.

Comments: